On detached potential flow and the d'Alembert's paradox (September-2022)
The Potential Flow Theory (PFT) states that a 'potential flow' must be incompressible (divergence-free) and irrotational (vorticity-free). However, compressible potential flow also exists, but it is not defined within the classical PFT. Then, strictly and in a broader sense, a potential flow must only fulfill the irrotationality condition. At this point, the term 'viscosity' is absent from such a definition, however, for the same reason that such a term does not appear, the fluid-flow viscosity value can be considered zero. Then, an 'inviscid potential flow' is now called an 'ideal flow' to be more specific. However, from the current interpretation, a viscous-forced potential flow (Kutta-Zhukovski-type pseudo-real flow) can also be defined, hence, 'potential flow' is not a synonym of ideal flow, as most authors misunderstand. In other words, the ideal flow is a potential flow, but a potential flow is not necessarily the ideal flow (see the next flowchart for better comprehension).
But, why if the ideal flow is by definition inviscid, its streamlines perfectly round an immersed body, as a two-dimensional cylinder, under the current theory/assumptions?
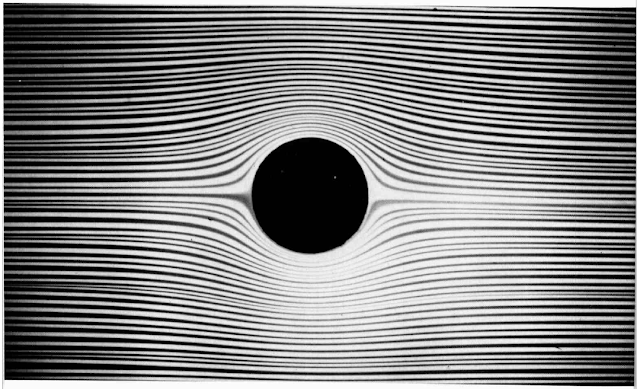
Zero viscosity should mean that shear stresses are absent between the body's surface and the flow's first layer, as well as between the remaining ones (layers). Then, what force causes the streamline to deform downstream from its undisturbed/straight path (after its hypothetical inviscid separation points at 90 and -90 deg.)? Perhaps the (steady) velocity field? However, the velocity field depends only on potential function (phi), which does not depend on viscosity, according to PFT. Even more, what would be the difference between such a streamlined pattern and another case considering an infinitely/large value for viscosity? The same, right? (See Fig. 2 and Fig. 3). At this point, there is an inconsistency, and the "paradox" seems to become a fallacy. Is this the reason to obtain theoretically zero drag (a nonphysical result) under such an attached flow assumption?
It should be remembered that, originally, the PFT was applied by M. Kutta and N. Zhukovski to justify the generation of the total (viscous + inviscid) lift component on an immersed body (e.g., a bidimensional thin flat plate), but not the (total) drag. In any case, only the induced drag is obtained by the projection of the lift vector. However, from such an approach, the viscosity is being imposed by attaching the flow to the plate (a viscous assumption, which is a clear contradiction within an inviscid approach!). Then, should this misnamed "ideal flow" be viscous (attached) or inviscid (detached)?! If such flow is considered inviscid (ideal), then vorticity should be detached from the sharp (zero thickness) leading edge, independently of the angle of attack, right? Finally, obtaining the true ideal flow result (a lower lift caused only by the inviscid contribution, within the low AoA range), which should be independent of the Reynolds number (see Fig. 4).
Finally, are the current assumptions of the classical PFT wrong?! No. It is only a particular case (a forced/imposed viscous version of potential flow, in fact!) of an "extended" theory, which should treat all flows as fully detached (true inviscid), being the fluid viscosity, an external factor to the original definition of a potential flow as said before, 'a' cause to maintain the flow attached to the body's surface; another one could be due to flow recirculation (with reattachment) behind the body/plate, caused by the unsteady-developed velocity field, a condition that should not be forced a priori through an embedded/attached/simplified circulation-vorticity model.
An alternate PFT could be explained through some potential numerical method, for example, based on the vortex lattice method (VLM), which has demonstrated that theoretical aspects behind a new hypothetical PFT remain the same as the original (most probably due to the principle of superposition), but only incorporate additional assumptions for detaching the flow [1].
By the way, why does a Stokes 'flow' (Re→0) have the same streamlined pattern as an "ideal" one (nu=0, hence Re→∞) described by the current interpretation of the Potential Flow Theory?
This text was originally written in September 2022 on the Researchgate.net portal but this has been adapted for this format.
[1] Journal's article: The Full Multi-wake Vortex Lattice Method: a detached flow model based on Potential Flow Theory | Advances in Aerodynamics | Full Text (springeropen.com)




Do u know prof. Taha's theory on lift? I think it could be compatible with your proposal..
ReplyDeleteYes, I found a paper last year, and I've seen some of his videos. Both approaches defend that lift force could exist even without fluid viscosity; thus, the Kutta condition doesn't have a viscous motivation (in fact, IMO, it's associated with a completely inviscid one!). However, I think that a correct reinterpretation of the existing Potential Flow Theory should be sufficient to solve some fundamental problems in fluid dynamics, including lift generation. Although it is also true that a problem can be solved in several ways, let's see what happens!
ReplyDeleteHi, it's not very clear to me how the Euler-D'Alembert paradox is a fallacy.
ReplyDeleteHi! I called it this way because such a description is trying to explain the inexistence of the drag force through a wrong assumption by forcing an "inviscid" flow to perfectly round the cylinder, as it was (almost) totally viscous (a totally viscous one will cause full stagnant flow without streamlines and then any force!).
DeleteAs we know from physical (not numerical...) experiments, since the Re increases, which inherently means that the viscosity effect decreases (or the characteristic length is relatively large), an inviscid or pure inertial condition is being reached, obtaining flow detachment with different patterns (symmetrical, von Karman, etc.). Thus, an ideal (true theoretical inviscid) flow condition must show a similar pattern but not an attached one, as in the current interpretation.
Thank you for the answer. Yes, of course the paradox is true only when its assumptions are (and in general they can hold true also for viscous fluids). The classical "solutions" of the paradox stems of course from the everyday experience of flows, and they rest on the boundary layer and/or wake concepts. The paradox is a fallacy in that it doesn't describe the reality we experience, not in the sense that its logic is flawed.
DeleteThis recent paper https://link.springer.com/article/10.1007/s00021-008-0290-1 also proposes a "solution" resting on instability and turbulence, which in a way is similar to what you said in the comment.
Thanks for the paper! There are some interesting results. The question here is: why have this (and other) "solutions" not been recognized as the true ones yet? Or maybe it is because it has not been applied to obtain practical solutions? I mean, maybe it does not matter to 'solve' the paradox, but what can be done with such knowledge...
DeleteThere is no "true" solution, as those solutions simply highlight features of the flow (boundary layer, wake, turbulence, instability), each on its own sufficient to invalidate the hypotheses of the paradox. These concepts have been applied since at least a century ago to obtain a lot of very practical results, even with "simplified" theories (vs. the Navier-Stokes equations).
Delete